Maximum-Weight Matching: Difference between revisions
Jump to navigation
Jump to search
No edit summary |
No edit summary |
||
| Line 10: | Line 10: | ||
== Parameters == | == Parameters == | ||
n: number of vertices | $n$: number of vertices | ||
m: number of edges | $m$: number of edges | ||
N: largest weight magnitude | $N$: largest weight magnitude | ||
== Table of Algorithms == | == Table of Algorithms == | ||
| Line 28: | Line 28: | ||
| [[Edmonds (Maximum-Weight Matching Maximum-Weight Matching)|Edmonds]] || 1965 || $O(mn^{2})$ || $O(mn^{2})$?? || Exact || Deterministic || [https://nvlpubs.nist.gov/nistpubs/jres/69B/jresv69Bn1-2p125_A1b.pdf Time] | | [[Edmonds (Maximum-Weight Matching Maximum-Weight Matching)|Edmonds]] || 1965 || $O(mn^{2})$ || $O(mn^{2})$?? || Exact || Deterministic || [https://nvlpubs.nist.gov/nistpubs/jres/69B/jresv69Bn1-2p125_A1b.pdf Time] | ||
|- | |- | ||
| [[Micali; Vazirani ( Maximum-Weight Matching)|Micali; Vazirani]] || 1980 || $O(n^{3} | | [[Micali; Vazirani ( Maximum-Weight Matching)|Micali; Vazirani]] || 1980 || $O(n^{3} \log n)$ || || Exact || Deterministic || [https://ieeexplore.ieee.org/document/4567800 Time] | ||
|- | |- | ||
| [[Mucha and Sankowski ( Maximum-Weight Matching)|Mucha and Sankowski]] || 2004 || $O(n^{3})$ || || Exact || Deterministic || [https://dl.acm.org/doi/10.1109/FOCS.2004.40 Time] | | [[Mucha and Sankowski ( Maximum-Weight Matching)|Mucha and Sankowski]] || 2004 || $O(n^{3})$ || || Exact || Deterministic || [https://dl.acm.org/doi/10.1109/FOCS.2004.40 Time] | ||
Revision as of 07:52, 10 April 2023
Description
In computer science, the maximum weight matching problem is the problem of finding, in a weighted graph, a matching in which the sum of weights is maximized. Here, the graph is unrestricted; i.e. can be any general graph.
Related Problems
Subproblem: Bipartite Maximum-Weight Matching
Parameters
$n$: number of vertices
$m$: number of edges
$N$: largest weight magnitude
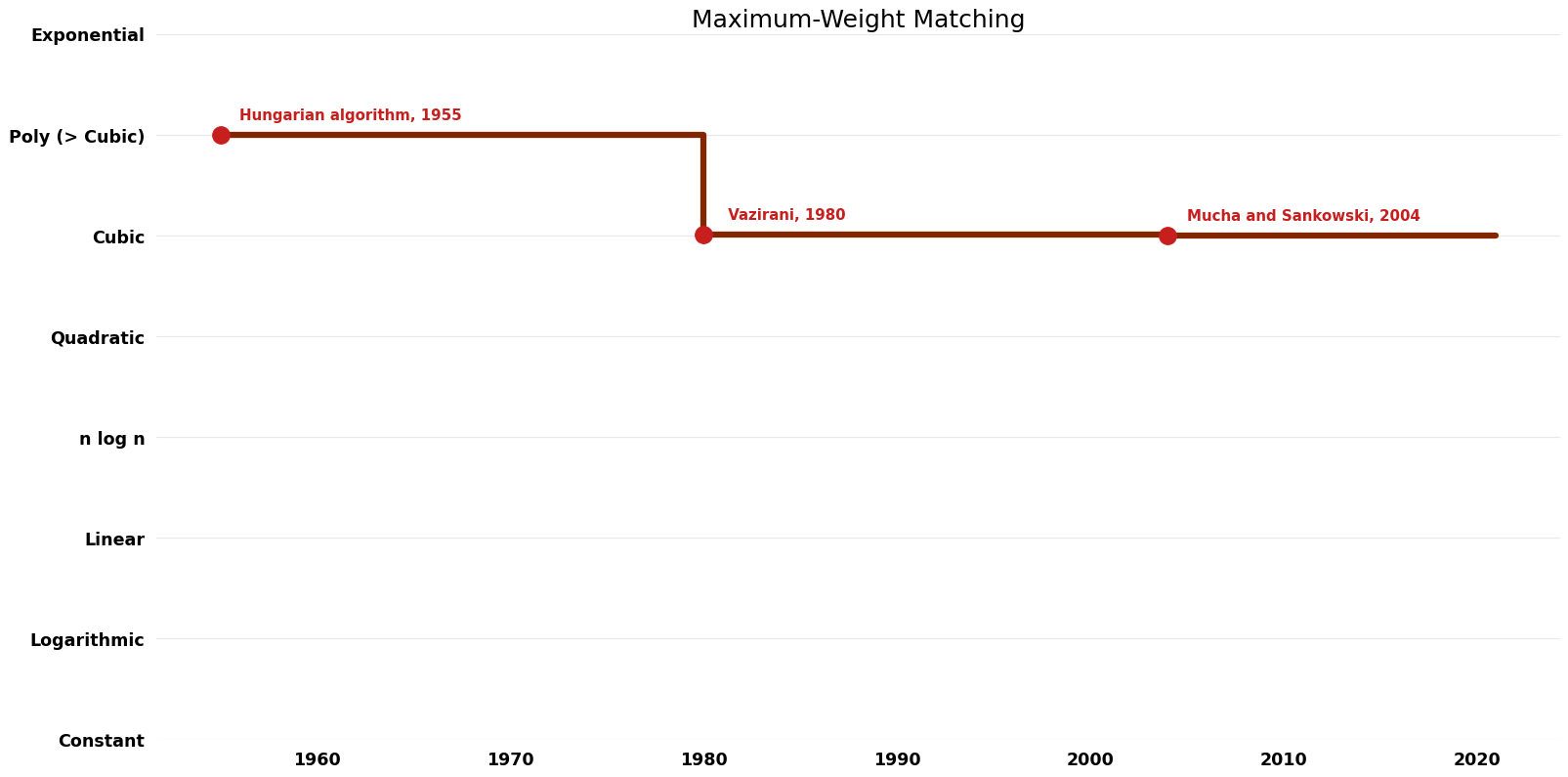
Table of Algorithms
| Name | Year | Time | Space | Approximation Factor | Model | Reference |
|---|---|---|---|---|---|---|
| Hungarian algorithm | 1955 | $O(n^{4})$ | $O(n^{2})$ | Exact | Deterministic | Time |
| Edmonds | 1965 | $O(mn^{2})$ | $O(mn^{2})$?? | Exact | Deterministic | Time |
| Micali; Vazirani | 1980 | $O(n^{3} \log n)$ | Exact | Deterministic | Time | |
| Mucha and Sankowski | 2004 | $O(n^{3})$ | Exact | Deterministic | Time |
Time Complexity Graph
Space Complexity Graph
Time-Space Tradeoff
